how to find the value of x in a right triangle

Learning Outcomes

• Use right triangles to evaluate trigonometric functions.
• Notice function values for 30° (π/6),   45° (π/4), and 60° (π/3).
• Use cofunctions of complementary angles.
• Use the definitions of trigonometric functions of any bending.
• Utilize right triangle trigonometry to solve applied problems.

Using Right Triangles to Evaluate Trigonometric Functions

In earlier sections, we used a unit circumvolve to ascertain the trigonometric functions. In this section, nosotros volition extend those definitions so that nosotros can utilize them to correct triangles. The value of the sine or cosine function of [latex]t[/latex] is its value at [latex]t[/latex] radians. First, we need to create our right triangle. Figure ane shows a signal on a unit circle of radius i. If we drib a vertical line segment from the signal [latex]\left(ten,y\correct)\\[/latex] to the ten-axis, nosotros accept a correct triangle whose vertical side has length [latex]y[/latex] and whose horizontal side has length [latex]x[/latex]. Nosotros can use this correct triangle to redefine sine, cosine, and the other trigonometric functions as ratios of the sides of a right triangle.

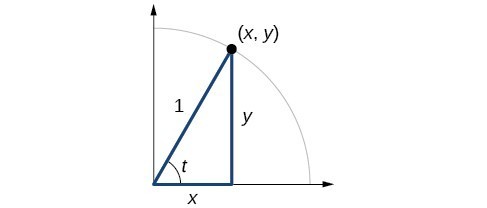
Effigy 1

We know

[latex]\cos t=\frac{x}{1}=ten[/latex]

Likewise, we know

[latex]\sin t=\frac{y}{1}=y[/latex]

These ratios still apply to the sides of a correct triangle when no unit circumvolve is involved and when the triangle is not in standard position and is not beingness graphed using [latex]\left(x,y\correct)[/latex] coordinates. To be able to use these ratios freely, we will give the sides more full general names: Instead of [latex]x[/latex], nosotros volition call the side between the given angle and the right bending the adjacent side to bending [latex]t[/latex]. (Side by side means "next to.") Instead of [latex]y[/latex], nosotros volition call the side almost distant from the given angle the reverse side from bending [latex]t[/latex]. And instead of [latex]one[/latex], we will phone call the side of a right triangle opposite the correct angle the hypotenuse. These sides are labeled in Figure 2.

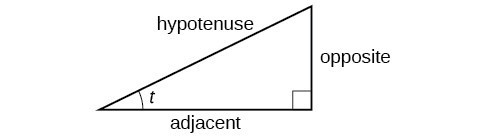
Figure 2.The sides of a right triangle in relation to angle [latex]t[/latex].

Understanding Right Triangle Relationships

Given a right triangle with an astute bending of [latex]t[/latex],

[latex]\begin{align}&\sin \left(t\right)=\frac{\text{opposite}}{\text{hypotenuse}} \\ &\cos \left(t\right)=\frac{\text{next}}{\text{hypotenuse}} \\ &\tan \left(t\right)=\frac{\text{opposite}}{\text{adjacent}} \end{align}[/latex]

A common mnemonic for remembering these relationships is SohCahToa, formed from the first letters of "Sine is opposite over hypotenuse, Cosine is adjacent over hypotenuse, Tangent is opposite over adjacent."

How To: Given the side lengths of a right triangle and 1 of the acute angles, notice the sine, cosine, and tangent of that angle.

1. Find the sine as the ratio of the reverse side to the hypotenuse.
2. Notice the cosine as the ratio of the adjacent side to the hypotenuse.
3. Find the tangent is the ratio of the reverse side to the adjacent side.

Example i: Evaluating a Trigonometric Function of a Right Triangle

Given the triangle shown in Figure 3, notice the value of [latex]\cos \alpha[/latex].

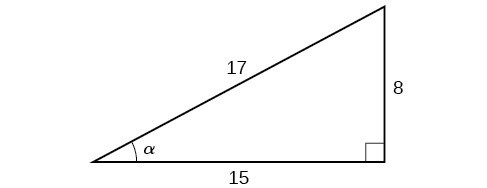
Figure 3

Attempt It

Given the triangle shown in Figure 4, find the value of [latex]\text{sin}t[/latex].

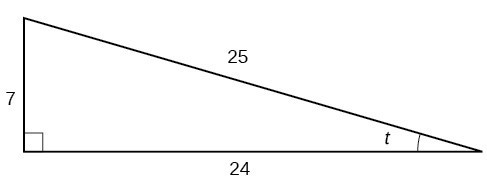
Effigy 4

Show Solution

[latex]\frac{7}{25}[/latex]

Relating Angles and Their Functions

When working with right triangles, the same rules employ regardless of the orientation of the triangle. In fact, we tin can evaluate the six trigonometric functions of either of the two acute angles in the triangle in Figure 5. The side opposite one acute angle is the side adjacent to the other astute bending, and vice versa.

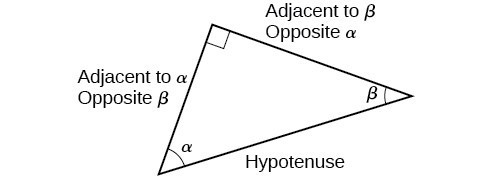
Figure five. The side adjacent to one angle is opposite the other.

We volition be asked to discover all half-dozen trigonometric functions for a given angle in a triangle. Our strategy is to find the sine, cosine, and tangent of the angles kickoff. Then, we tin discover the other trigonometric functions hands because we know that the reciprocal of sine is cosecant, the reciprocal of cosine is secant, and the reciprocal of tangent is cotangent.

How To: Given the side lengths of a right triangle, evaluate the 6 trigonometric functions of i of the acute angles.

1. If needed, draw the correct triangle and label the angle provided.
2. Place the angle, the adjacent side, the side reverse the bending, and the hypotenuse of the correct triangle.
3. Find the required office:
   • sine every bit the ratio of the opposite side to the hypotenuse
   • cosine equally the ratio of the next side to the hypotenuse
   • tangent as the ratio of the opposite side to the side by side side
   • secant as the ratio of the hypotenuse to the adjacent side
   • cosecant every bit the ratio of the hypotenuse to the opposite side
   • cotangent equally the ratio of the adjacent side to the contrary side

Instance 2: Evaluating Trigonometric Functions of Angles Not in Standard Position

Using the triangle shown in Figure 6, evaluate [latex]\sin \alpha[/latex], [latex]\cos \alpha[/latex], [latex]\tan \alpha[/latex], [latex]\sec \alpha[/latex], [latex]\csc \alpha [/latex], and [latex]\cot \blastoff[/latex].

Figure vi

Bear witness Solution

[latex]\begin{align}&\sin \alpha =\frac{\text{opposite }\alpha }{\text{hypotenuse}}=\frac{iv}{five}\\ &\cos \alpha =\frac{\text{side by side to }\alpha }{\text{hypotenuse}}=\frac{3}{five} \\ &\tan \alpha =\frac{\text{opposite }\blastoff }{\text{adjacent to }\alpha }=\frac{4}{3} \\ &\sec \blastoff =\frac{\text{hypotenuse}}{\text{side by side to }\blastoff }=\frac{5}{3} \\ &\csc \blastoff =\frac{\text{hypotenuse}}{\text{opposite }\blastoff }=\frac{five}{4} \\ &\cot \alpha =\frac{\text{next to }\blastoff }{\text{opposite }\blastoff }=\frac{3}{4} \terminate{align}[/latex]

Try It

Using the triangle shown in Figure 7, evaluate [latex]\sin t[/latex], [latex]\cos t[/latex], [latex]\tan t[/latex], [latex]\sec t[/latex], [latex]\csc t[/latex], and [latex]\cot t[/latex].

Effigy 7

Show Solution

[latex]\begin{align}&\sin t=\frac{33}{65},\cos t=\frac{56}{65},\tan t=\frac{33}{56}, \\ &\sec t=\frac{65}{56},\csc t=\frac{65}{33},\cot t=\frac{56}{33} \end{align}[/latex]

Try It

Finding Trigonometric Functions of Special Angles Using Side Lengths

Nosotros accept already discussed the trigonometric functions equally they chronicle to the special angles on the unit circle. Now, we can use those relationships to evaluate triangles that comprise those special angles. We do this because when nosotros evaluate the special angles in trigonometric functions, they have relatively friendly values, values that contain either no or simply one square root in the ratio. Therefore, these are the angles often used in math and science problems. We will utilise multiples of [latex]30^\circ [/latex], [latex]sixty^\circ [/latex], and [latex]45^\circ[/latex], even so, remember that when dealing with right triangles, we are limited to angles between [latex]0^\circ \text{ and } 90^\circ[/latex].

Suppose we have a [latex]thirty^\circ ,sixty^\circ ,xc^\circ [/latex] triangle, which tin as well exist described as a [latex]\frac{\pi }{six}, \frac{\pi }{3},\frac{\pi }{2}[/latex] triangle. The sides take lengths in the relation [latex]s,\sqrt{3}south,2s[/latex]. The sides of a [latex]45^\circ ,45^\circ ,90^\circ [/latex] triangle, which can also be described as a [latex]\frac{\pi }{4},\frac{\pi }{four},\frac{\pi }{2}[/latex] triangle, take lengths in the relation [latex]due south,s,\sqrt{2}s[/latex]. These relations are shown in Figure 8.

Figure 8. Side lengths of special triangles

We can so use the ratios of the side lengths to evaluate trigonometric functions of special angles.

How To: Given trigonometric functions of a special angle, evaluate using side lengths.

1. Utilize the side lengths shown in Figure viii for the special bending y'all wish to evaluate.
2. Employ the ratio of side lengths appropriate to the function you wish to evaluate.

Example 3: Evaluating Trigonometric Functions of Special Angles Using Side Lengths

Find the exact value of the trigonometric functions of [latex]\frac{\pi }{3}[/latex], using side lengths.

Show Solution

[latex]\begin{align}&\sin \left(\frac{\pi }{3}\correct)=\frac{\text{opp}}{\text{hyp}}=\frac{\sqrt{three}s}{2s}=\frac{\sqrt{3}}{2}\\ &\cos \left(\frac{\pi }{three}\right)=\frac{\text{adj}}{\text{hyp}}=\frac{s}{2s}=\frac{1}{2}\\ &\tan \left(\frac{\pi }{3}\right)=\frac{\text{opp}}{\text{adj}}=\frac{\sqrt{3}s}{s}=\sqrt{3}\\ &\sec \left(\frac{\pi }{3}\correct)=\frac{\text{hyp}}{\text{adj}}=\frac{2s}{due south}=two\\ &\csc \left(\frac{\pi }{3}\right)=\frac{\text{hyp}}{\text{opp}}=\frac{2s}{\sqrt{3}due south}=\frac{2}{\sqrt{3}}=\frac{2\sqrt{3}}{three} \\ &\cot \left(\frac{\pi }{3}\right)=\frac{\text{adj}}{\text{opp}}=\frac{s}{\sqrt{three}s}=\frac{one}{\sqrt{3}}=\frac{\sqrt{iii}}{iii} \finish{align}[/latex]

Try Information technology

Detect the exact value of the trigonometric functions of [latex]\frac{\pi }{4}[/latex], using side lengths.

Prove Solution

[latex]\sin \left(\frac{\pi }{4}\right)=\frac{\sqrt{2}}{2},\cos \left(\frac{\pi }{iv}\right)=\frac{\sqrt{two}}{2},\tan \left(\frac{\pi }{iv}\right)=1[/latex],
[latex]\sec \left(\frac{\pi }{four}\right)=\sqrt{two},\csc\left(\frac{\pi }{4}\correct)=\sqrt{two},\cot \left(\frac{\pi }{4}\correct)=1[/latex]

Using Equal Cofunction of Complements

If we look more closely at the human relationship between the sine and cosine of the special angles relative to the unit circle, nosotros volition notice a pattern. In a correct triangle with angles of [latex]\frac{\pi }{six}[/latex] and [latex]\frac{\pi }{3}[/latex], nosotros run into that the sine of [latex]\frac{\pi }{3}[/latex], namely [latex]\frac{\sqrt{3}}{ii}[/latex], is also the cosine of [latex]\frac{\pi }{6}[/latex], while the sine of [latex]\frac{\pi }{half-dozen}[/latex], namely [latex]\frac{ane}{2}[/latex], is also the cosine of [latex]\frac{\pi }{3}[/latex].

[latex]\begin{align} &\sin \frac{\pi }{3}=\cos \frac{\pi }{6}=\frac{\sqrt{3}s}{2s}=\frac{\sqrt{iii}}{2} \\ &\sin \frac{\pi }{6}=\cos \frac{\pi }{3}=\frac{s}{2s}=\frac{one}{two} \end{align}[/latex]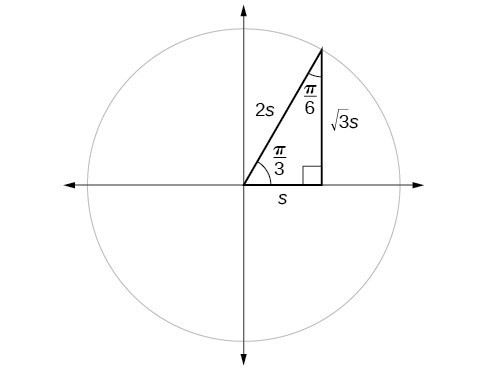

Figure nine.The sine of [latex]\frac{\pi }{3}[/latex] equals the cosine of [latex]\frac{\pi }{6}[/latex] and vice versa.

This result should non be surprising because, as we meet from Figure 9, the side contrary the angle of [latex]\frac{\pi }{3}[/latex] is also the side adjacent to [latex]\frac{\pi }{6}[/latex], so [latex]\sin \left(\frac{\pi }{3}\right)[/latex] and [latex]\cos \left(\frac{\pi }{6}\correct)[/latex] are exactly the aforementioned ratio of the aforementioned two sides, [latex]\sqrt{3}s[/latex] and [latex]2s[/latex]. Similarly, [latex]\cos \left(\frac{\pi }{three}\right)[/latex] and [latex]\sin \left(\frac{\pi }{vi}\right)[/latex] are too the same ratio using the same 2 sides, [latex]s[/latex] and [latex]2s[/latex].

The interrelationship betwixt the sines and cosines of [latex]\frac{\pi }{half dozen}[/latex] and [latex]\frac{\pi }{three}[/latex] also holds for the two acute angles in whatever correct triangle, since in every case, the ratio of the same two sides would constitute the sine of one bending and the cosine of the other. Since the three angles of a triangle add together to [latex]\pi [/latex], and the right angle is [latex]\frac{\pi }{2}[/latex], the remaining ii angles must as well add up to [latex]\frac{\pi }{2}[/latex]. That means that a right triangle can be formed with any ii angles that add together to [latex]\frac{\pi }{2}[/latex] —in other words, whatsoever two complementary angles. So nosotros may state a cofunction identity: If any two angles are complementary, the sine of one is the cosine of the other, and vice versa. This identity is illustrated in Figure ten.

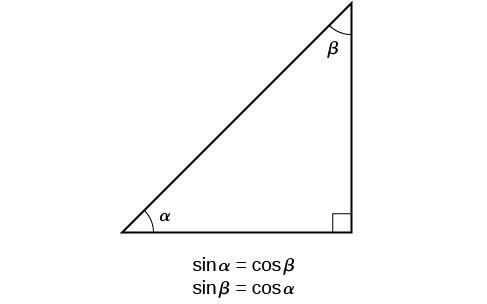
Figure x.Cofunction identity of sine and cosine of complementary angles

Using this identity, nosotros can land without calculating, for case, that the sine of [latex]\frac{\pi }{12}[/latex] equals the cosine of [latex]\frac{v\pi }{12}[/latex], and that the sine of [latex]\frac{5\pi }{12}[/latex] equals the cosine of [latex]\frac{\pi }{12}[/latex]. We can also state that if, for a certain angle [latex]t[/latex], [latex]\cos \text{ }t=\frac{5}{13}[/latex], then [latex]\sin \left(\frac{\pi }{2}-t\correct)=\frac{5}{xiii}[/latex] as well.

A General Note: Cofunction Identities

The cofunction identities in radians are listed in the table below.

[latex]\cos t=\sin \left(\frac{\pi }{two}-t\right)[/latex]	[latex]\sin t=\cos \left(\frac{\pi }{2}-t\right)[/latex]
[latex]\tan t=\cot \left(\frac{\pi }{two}-t\correct)[/latex]	[latex]\cot t=\tan \left(\frac{\pi }{2}-t\right)[/latex]
[latex]\sec t=\csc \left(\frac{\pi }{2}-t\right)[/latex]	[latex]\csc t=\sec \left(\frac{\pi }{2}-t\right)[/latex]

How To: Given the sine and cosine of an bending, find the sine or cosine of its complement.

1. To find the sine of the complementary angle, find the cosine of the original angle.
2. To detect the cosine of the complementary angle, detect the sine of the original bending.

Case 4: Using Cofunction Identities

If [latex]\sin t=\frac{v}{12}[/latex], detect [latex]\cos \left(\frac{\pi }{2}-t\right)[/latex].

Effort It

If [latex]\csc \left(\frac{\pi }{vi}\correct)=ii[/latex], find [latex]\sec \left(\frac{\pi }{3}\right)[/latex].

Using Trigonometric Functions

In previous examples, we evaluated the sine and cosine in triangles where nosotros knew all three sides. But the real ability of correct-triangle trigonometry emerges when we await at triangles in which we know an bending only practice not know all the sides.

How To: Given a right triangle, the length of one side, and the measure of i acute angle, find the remaining sides.

1. For each side, select the trigonometric office that has the unknown side as either the numerator or the denominator. The known side will in turn be the denominator or the numerator.
2. Write an equation setting the role value of the known angle equal to the ratio of the respective sides.
3. Using the value of the trigonometric office and the known side length, solve for the missing side length.

Instance 5: Finding Missing Side Lengths Using Trigonometric Ratios

Find the unknown sides of the triangle in Figure xi.

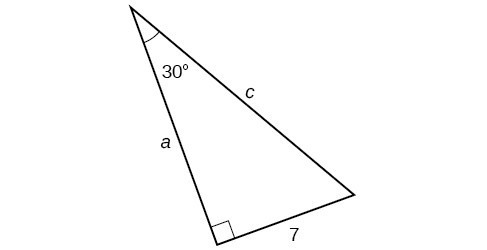
Figure eleven

Attempt It

A right triangle has one angle of [latex]\frac{\pi }{3}[/latex] and a hypotenuse of 20. Detect the unknown sides and angle of the triangle.

Show Solution

[latex]\text{next}=10[/latex]; [latex]\text{reverse}=10\sqrt{3}[/latex] ; missing angle is [latex]\frac{\pi }{half dozen}[/latex]

Attempt It

Correct-triangle trigonometry has many practical applications. For case, the ability to compute the lengths of sides of a triangle makes information technology possible to notice the top of a alpine object without climbing to the acme or having to extend a tape measure out along its height. We do and so by measuring a distance from the base of the object to a point on the ground some distance away, where nosotros can look up to the top of the alpine object at an angle. The bending of elevation of an object higher up an observer relative to the observer is the angle between the horizontal and the line from the object to the observer's eye. The right triangle this position creates has sides that represent the unknown tiptop, the measured altitude from the base, and the angled line of sight from the ground to the tiptop of the object. Knowing the measured distance to the base of the object and the angle of the line of sight, we can use trigonometric functions to calculate the unknown top. Similarly, we can class a triangle from the top of a tall object by looking downward. The angle of depression of an object beneath an observer relative to the observer is the angle between the horizontal and the line from the object to the observer's middle.

Figure 12

How To: Given a tall object, measure out its summit indirectly.

1. Make a sketch of the problem state of affairs to go on rail of known and unknown information.
2. Lay out a measured altitude from the base of the object to a point where the top of the object is clearly visible.
3. At the other end of the measured distance, look up to the superlative of the object. Measure the angle the line of sight makes with the horizontal.
4. Write an equation relating the unknown height, the measured distance, and the tangent of the bending of the line of sight.
5. Solve the equation for the unknown elevation.

Example 6: Measuring a Distance Indirectly

To observe the height of a tree, a person walks to a indicate 30 anxiety from the base of the tree. She measures an bending of [latex]57^\circ [/latex] between a line of sight to the elevation of the tree and the ground, as shown in Figure thirteen. Detect the acme of the tree.

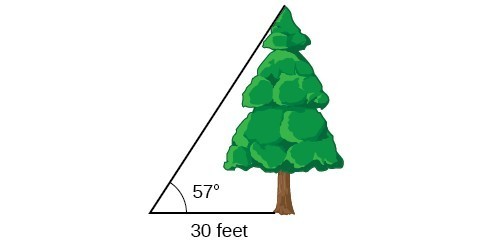
Figure 13

Endeavor It

How long a ladder is needed to reach a windowsill 50 feet higher up the basis if the ladder rests against the building making an angle of [latex]\frac{5\pi }{12}[/latex] with the footing? Round to the nearest foot.

Testify Solution

About 52 ft

Endeavour Information technology

Key Equations

Cofunction Identities

[latex]\begin{gathered} \cos t=\sin \left(\frac{\pi }{2}-t\correct) \\ \sin t=\cos \left(\frac{\pi }{ii}-t\right)\\ \tan t=\cot \left(\frac{\pi }{2}-t\right) \\ \cot t=\tan \left(\frac{\pi }{two}-t\right) \\ \sec t=\csc \left(\frac{\pi }{two}-t\correct) \\ \csc t=\sec \left(\frac{\pi }{ii}-t\right) \end{gathered}[/latex]

Key Concepts

• We can define trigonometric functions every bit ratios of the side lengths of a right triangle.
• The same side lengths can be used to evaluate the trigonometric functions of either acute angle in a right triangle.
• We tin evaluate the trigonometric functions of special angles, knowing the side lengths of the triangles in which they occur.
• Any two complementary angles could be the two acute angles of a correct triangle.
• If ii angles are complementary, the cofunction identities state that the sine of one equals the cosine of the other and vice versa.
• Nosotros tin can use trigonometric functions of an bending to find unknown side lengths.
• Select the trigonometric function representing the ratio of the unknown side to the known side.
• Right-triangle trigonometry permits the measurement of inaccessible heights and distances.
• The unknown height or distance tin can be plant by creating a right triangle in which the unknown height or altitude is one of the sides, and another side and angle are known.

Glossary

adjacent side

in a correct triangle, the side between a given bending and the correct angle

angle of low

the bending between the horizontal and the line from the object to the observer'due south centre, assuming the object is positioned lower than the observer

angle of elevation

the angle betwixt the horizontal and the line from the object to the observer's heart, bold the object is positioned college than the observer

opposite side

in a right triangle, the side near distant from a given angle

hypotenuse

the side of a correct triangle opposite the right angle

Source: https://courses.lumenlearning.com/precalculus/chapter/right-triangle-trigonometry/

Posted by: givenslithad.blogspot.com

Share this post